The delayed-choice quantum eraser experiment does not rewrite the past
There’s a mind-bending physics experiment that suggests to many people that the past can be rewritten. A choice in the experiment appears to affect a measurement but the choice is made after the measurement is recorded. The future choice appears to determine the past measurement (hence, delayed-choice).

Changing the past!? Retrocausality!? Who would believe such a thing!? Well… me. I wasn’t alone. It seems that 99% of people who come across videos and blogs about the experiment online seem to come away thinking the past can be rewritten due to the weirdness of quantum mechanics, or at the very least we’ve got quantum mechanics wrong if it says retrocausality is taking place. I’m already able to accept seemingly wild ideas like eternalism and an Everetian approach to quantum mechanics so why the hell not? If there’s one thing quantum mechanics teaches us, it’s that the world is very different than our daily experiences suggest. If the science suggests that retrocausality exists then I’ll take it seriously, and I did for many years.
Almost every video, blog post, or article I’ve ever seen about the experiment has made the retrocausality claim but there are a tiny minority that make the case for it being a misunderstanding. I watched and read those cases and wasn’t convinced. Sometimes I didn’t really get the point, sometimes I didn’t think they were right, and again I wasn’t alone. The comments were always full of people saying “I’m even more confused now” or “this seems very different from the actual experiment, I don’t think you’re right”. Some worthy attempts to explain what’s going on but they didn’t stick the landing for me and seemingly many others.
I’m no longer convinced there’s any retrocausality happening and I don’t think the confusion comes from quantum weirdness either. It’s just people misunderstanding the experiment, being attracted to patterns regardless of origin, and mixing up correlation and causation. As someone who bought into the weirdness of the experimental results, didn’t understand the counterclaims, and now gets that there’s nothing weird at all, I’d like to try explaining. I’m borrowing ideas from others but I want to present it differently in order to emphasise the parts that helped me and to keep it as simple as possible.
Simplifying the experiment
The experiment reveals some weirdness of quantum mechanics like the idea of partial decoherence but the retrocausality (future causing events in the past) emerges from an aspect of the experiment that is totally classical (non-quantum). As suggested in this video by Craig Gidney, you can make a classical version of the experiment, with no quantum weirdness, and still get supposed retrocausality in the results. It’s worth using a classical analog of the experiment to demonstrate the non-quantum nature of the problem but also because it becomes easier to spot where the misunderstanding is.
Firstly, let’s describe the real experiment but simplify it in a diagram. Much of its complexity arises from the fact that it’s a difficult experiment to actually perform in the real world for technical reasons but we only need the key details. It starts with a process that creates a correlation, in this case through entanglement (which is correlation on steroids). You can think of the experiment as using entangled photons or a superposition of paths or being more honest and sticking with a wave through the whole system but for simplicity let’s just say entangled photons. This means they are correlated so measuring one tells you something about the other.
Technical detail: In the experiment, the light goes through a double-slit first before creating two entangled photons so using a classical-leaning, particle-centric language we can say that if we learn one photon went through the left slit, we know the other photon did too or vice versa. The important point is they are correlated and knowing the state of one tells you something about the state of the other.
We begin by creating a correlation through entanglement:

Next we send the photons out to detectors but the top photon arrives at a detector first. The bottom photon travels further and has a chance of either being detected in such a way that the correlation remains (the first detector) or in a way that will mess with our correlation (the second detector). The exact experimental setup creates what is called a quantum eraser that is essentially removing the knowledge we have of the correlation.
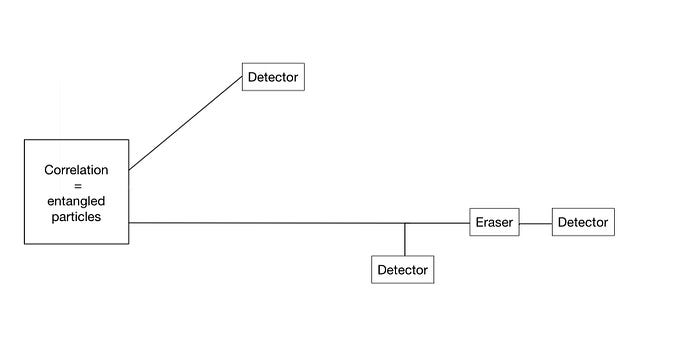
The bottom photon has a chance of being at the detector before or after the eraser. It’s important to note that this “choice” happens after the top photon has hit the top detector.
For simplicity I’ve drawn the diagram to show only 2 detectors that either detect the photon before or after the eraser because that’s the “choice” and all that’s important for the point I’m making. Technically there are two detectors on each side of the eraser because that’s what tells you if the photon “took” the left or right slit but right now we’re focusing on the fact that there’s a choice of whether it’s the first detectors before the eraser or the next detectors after the eraser (in the full experiment, the final detectors and the way they’re set up ARE the eraser). We’re only interested in the fact that detections either happen before or after the eraser, which is the “choice” in the experiment as you don’t know in advance if the eraser will be used or not. This is the choice that will supposedly rewrite the past.
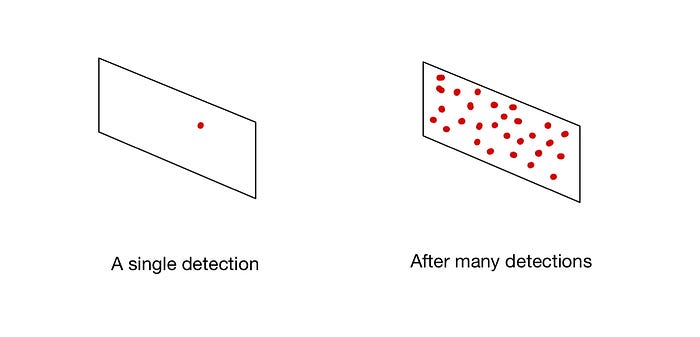
You can think of the detectors like the wall or screen you see at the back of a double-slit experiment. This experiment isn’t very useful to run just once. You would only get a single dot on the top detector and it wouldn’t tell you much. Instead, the experiment is run over and over like in the double-slit experiment where after a long time you may see a pattern emerge. Enjoy my art:
If we want to compare what happens at the top detector with what happens at the bottom detectors we need to be able to associate the dots on top with the dots on the bottom. We need to know which “choice” (i.e. did it go through the eraser or not) corresponds with which dot on the top detector. A coincidence circuit is added at the end so we don’t count random photons hitting the detectors and so we can look at specific runs of the experiment later. We need to be able to match partner photons at the end of the day.

Before we rebuild a classical version of the experiment it’s very important to understand a common misconception about the detectors. In most explanations you will see claims that detections at the bottom pre-eraser detector results in a certain pattern on the top detector and detections at the post-eraser detector will result in a different pattern at the top detector. Here’s an example from a PBS video showing a pattern on an interference screen (our top detector):

This is misleading. The top detector ALWAYS shows a smooth smattering of random dots. Always. Yes, I mean always. Nobody ever runs this experiment over and over and sees an interference pattern or banded pattern emerge on the screen. It’s always a smooth, random smattering of hits. People creating these videos aren’t deliberately misleading you; it’s just a shorthand way of talking about the final results that will be produced in later steps. Once all the data has been collected, we can filter the mess of top photons and say “only show me the hits that correspond with the eraser being used” and “only show me the hits that correspond with the eraser not being used” and they will show two different patterns. An interference pattern emerges out of the data if we look at the photons who had a partner detected after going through the eraser. A different pattern emerges for the top photons whose partners were detected before the eraser.
To summarise, the claim is that the photons hitting the top detector form different patterns based on whether or not the bottom photon was detected before or after the eraser. The eraser is essentially a choice but it’s a choice made after the top photon has already been detected. So how does the top photon know where to land and in order to form a specific pattern? How does it know what pattern to form? Does it know the future of its entangled partner? Does the observer (the scientist) learning of the bottom detector results cause history to rewrite itself so that the matching pattern appears in the earlier top detector? It seems so! Quantum weirdness strikes again! However, before we get carried away let’s try rebuilding the same experiment with no quantum effects involved. A correlation, a measurement, a later measurement involving a choice, and being able to corroborate between top and bottom hits at the end.
A delayed-choice CLASSICAL eraser experiment
Let’s rebuild the experiment with a classical analog based on the one described in the previously mentioned video. Entanglement is a form of correlation, so we can recreate the beginnings of the experiment. Instead of two entangled photons, I’m going to stand at the start and make sure that a die and coin match in oddness and evenness. Heads and tails on the coin can represent even and odd numbers so let’s simplify it further by just writing “even” and “odd” on the sides. From now on we can refer to the coin as landing either odd or even, rather than heads or tails.
I shake the die, check to see if it’s an even or odd number, and then correlate the two objects by placing the coin to show the matching property facing up. If a 1 shows on the die, I place the coin to show “odd” face up. The two objects begin the experiment correlated. Even or odd is really just an analog of the quantum experiment’s left slit or right slit but that’s not important. The point is by starting with a correlation, learning the state of one tells you about the other.

Next we need the top path with a top detector and this will be Alice. She received the die very carefully and notes the number. Her list of recorded results is just like the back wall of the top detector in the quantum experiment. Every run of the experiment she will have another number. Here she is after 12 runs.

Now we need to recreate the lower detectors so we send the coin to Bob. We’ll create a delayed choice by making sure Bob has a choice to make that takes place after Alice has already written down the result she got on our die. For example, if this experiment runs once every day then maybe Alice gets the die at 9am but Bob gets the coin at 9pm and we do this everyday.
In the quantum experiment we had detectors before and after the eraser because there was a chance the photon would go to the first one or go on to the eraser and therefore the next detector. We’ll let Bob make the choice himself by carefully handing him the coin in a box and letting him either open it carefully or shake it first before checking the coin and noting the result. Whether Bob opens and records it or shakes it first then records it is a choice that will take place after Alice has already recorded her result for the coin’s partner die.

We have an analog of the coincidence counter because Alice and Bob kept good notes and we know the order of each hit. If you want to know what Alice got when Bob made a certain choice, we can look at the data and figure it out just like in the quantum version. Not only does Bob note whether he got even or odd (heads or tails); he also notes whether he shook the box or not. We have similar information in the quantum experiment because which detector is hit tells us if the eraser was used or not (was the photon detected at a detector before or after the quantum eraser setup has an effect?).
Bob rewrites time
If we run the classical experiment, something amazing happens. It appears that different patterns emerge in Alice’s results depending on what choice Bob makes. Let’s demonstrate first using a language similar to how people describe the quantum experiment. Later, we’ll reconsider using different words and see if that changes your perspective.
Bob ultimately has 2 choices and 4 possible results because he notes whether or not he shook the box and what the result was.
- Didn’t shake the box and coin was even
- Didn’t shake the box and coin was odd
- Shook the box and coin was even
- Shook the box and coin was odd
We’ve already said that Alice always has a big, random, smattering of results but we can filter those results to show us specific conditions. We’re interested in the choice Bob had (to shake the box or not) because it was a choice made after Alice had recorded her result each time so let’s see the results Alice recorded when Bob didn’t shake the box.
Alice’s results when Bob chose not to shake the box and the coin was even:

Alice’s results when Bob chose not to shake the box and coin was odd:

Wow, this is already interesting. A banded pattern! Even though the results were a big random smattering of die values, a banded pattern is emerging from the data. Weird!
What about when Bob does shake the box first?
Alice’s results when Bob chose to shake the box and the coin was even:
Alice’s results when Bob chose to shake the box and coin was odd:

Now this is where the real weirdness shows up. A different pattern emerges in the data depending on whether or not Bob shook the box. This seems bizarre. Alice’s results have a specific pattern if Bob does one thing and a lack of that pattern if Bob does another thing, even though Bob’s choice about that thing was after Alice committed the result. This suggests that Alice’s die knew what Bob was going to do in advance or perhaps Bob’s choice caused past events to rewrite themselves to create the right pattern. Does the die know what number it will need to be to form the correct pattern?
What the heck is going on?
Hopefully you can see that Bob’s choice rewriting history is very similar to the original version of the experiment where quantum weirdness supposedly is the cause for the retrocausality. In the quantum experiment, different patterns emerge from the results depending on whether we’re filtering on detections made before or after the eraser. But is quantum weirdness really to blame when we see the same thing happening in a classical analog of the experiment? Alice’s results have different patterns depending on Bob’s choice after all.
The complexity of the quantum experiment and our unfamiliarity with concepts like coincidence counters lead us astray. We’re also misled by the use of imagery that suggests the top detector actually shows patterns itself as mentioned earlier. The point of this post is to show how I eventually came to understand what’s going on and improve on previous attempts at describing why the classical version supposedly features retrocausality. I’d like to do this by describing Alice and Bob’s results again but this time let’s change the words we use to better describe what’s really happening.
We said Bob had the following 4 possible outcomes because of 2 choices:
- Didn’t shake the box and coin was even
- Didn’t shake the box and coin was odd
- Shook the box and coin was even
- Shook the box and coin was odd
The most important thing about this whole explanation is that this isn’t the same kind of choice as apples or oranges, black or white, yes or no, and it isn’t even as simple as shake or don’t shake. The die and coin were correlated to begin with, which means you had useful information: the two objects match. If you know something about one, you then know something about the other. This is meaningful. Bob’s choice is actually whether he wants useful information or useless information. Does he want to maintain that correlation that tells him something, or lose it? We can rewrite those 4 possible outcomes again like this:
- The 2 objects definitely match and the coin was even
- The 2 objects definitely match and the coin was odd
- The 2 objects are no longer correlated and the coin was even
- The 2 objects are no longer correlated and the coin was odd
It might seem like a small thing but let’s run through the results again while focusing on what’s actually being asked when filtering. Alice has a big random smattering of results. We’re interested in what happens when Bob doesn’t shake the box but consider how we rewrote that request: the objects match.
“Hey Alice, show me what you got when the objects matched and I got even”

“Hey Alice, show me what you got when the objects matched and I got odd”

Are you still as surprised or impressed by a pattern emerging from the data? “When we know the objects matched and I got even, what did you get? Even as well!? Wow! That’s a surprising result! A banded pattern of only even results? Incredible. What about when we know they both matched and I got odd, what did you get? You also got odd? Wow. A different banding pattern. Who could have guessed our matching objects would match? That’s bizarre. It’s like your results knew what to be.” And of course they did know what to be. They were correlated at the beginning. It’s not surprising or amazing that when the die and coin matched in oddness and evenness that they were both odd or even. Bob is forcing a pattern out of the randomness of Alice’s results by filtering only to those where he knows they’ll match and he knows what he got. And what about shaking the box?
“Hey Alice, show me what you got when the coin no longer definitely matched the die and I got even”

“Hey Alice, show me what you got when the coin no longer definitely matched the die and I got odd”

Hopefully this is less impressive as well. When the die is randomly rolled at the start, and then the coin is randomly flipped by Bob (no longer correlated with the die), the results are random. But when we ask to see only the results where we know there will be a match, there is a match and it isn’t random. This occurs because we’re starting with a correlation, then the choice being made is to preserve useful information or lose it, then we’re filtering the full results to only show us those matches.
Compare these two different ways of describing the same request:
- Show me the results you got when I made choice x instead of choice y
- Show me the results you got that match my results as opposed to the results that don’t
If you want to describe Bob’s choice as transparently and meaningfully as possible, it would be “do I want information I could use in filtering later or none so my filtering will be meaningless later?” rather than “do I want to shake it or not”. The choice is about how you will be able to filter data and the shocking results are the filtering of data. When he chose to have a meaningful way to filter the data, he was able to filter the data meaningfully. When he chose not to have a meaningful way to filter the data, he wasn’t able to filter the data meaningfully. That’s it.
Bob isn’t really rewriting the past. He’s filtering Alice’s results in a way that’s difficult to follow because it’s after the fact, it’s essentially using a coincidence counter, and it’s not any random two-way choice but a decision to maintain or lose the correlation. Bob is choosing to keep info about what’s going on or have no idea what’s going on and in the end that’s exactly what the filtering shows. The same thing happens in the quantum version of the experiment because it starts with a correlation, there’s a decision to have useful or useless information, and then the results are filtered on whether they’re useful or not.
A classic(al) mistake
Hopefully you’ve followed along here but even if you can’t quite see why the patterns emerge one way and not the other, hopefully you can see that whatever the cause of the supposed retrocausality is it can’t be a quantum effect. This isn’t because of the weirdness of superpositions, entanglement, double-slits, decoherence etc. Even with a die and a coin we can have banded or smooth patterns emerge from the data when Bob’s decision came after Alice’s recording.
So why do we keep making this mistake? Why did I not see this before? Why couldn’t I see this even after coming across videos and posts explaining most of what I have here? What obscures the reality to the point that we think we’re rewriting the past? I believe these are the culprits:
- Telling people that quantum mechanics is weird. The delayed choice quantum eraser experiment was proposed in the 1970s and physicists could predict what would happen if the experiment could be performed. Decades later physicists had the technology to create the real experiment and the results were as predicted. When scientists use theories and models to understand the world, then make successful predictions, it usually means something is being understood. The results of the experiment aren’t weird. Many features of quantum mechanics seem weird to some but are mundane to physicists and understood well enough to be used in engineering and technology. The big mystery is explaining exactly how more fundamental physics maps onto the everyday physics you experience in daily life. Physicists debate exactly what quantum mechanics is doing in the background but the experiment and results make sense. Telling people it’s all weird and mystifying sets people up to believe anything weird. Telling people to take the Copenhagen interpretation seriously doesn’t help either.
- Suggesting the supposed retrocausality is related to quantum mechanics at all. There are quantum effects in the experiment but they aren’t related to the misunderstanding that causes people to believe retrocausality is occuring. The rewriting of the past is presented as being a quality that only emerges because this is a quantum experiment but a classical analog of the experiment would cause the same supposed retrocausality. This isn’t about quantum mechanics.
- The complexity of the experiment. The main phenomena that’s causing us to confuse correlation for causation here doesn’t require the experiment to be as complex as it is. We can simplify the experiment considerably and maintain the supposed retrocausality, even to the point of removing all quantum features as mentioned above. It’s important to realise that the original quantum experiment is very difficult to perform in the real world, which is why it was first performed decades after it was thought up. The real experiment has more steps and equipment that is required conceptually but is just a consequence of the technology we have and what we’re able to do. The complexity of the experiment, involving waves and particles and slits and entanglement and crystals and mirrors and erasers and detectors and coincidence counters, obscures the nature of what’s going on when the results are provided.
- Suggesting the patterns emerge on the detectors themselves. It’s not the main misconception behind the misunderstanding but people are definitely misled by imagery showing patterns appearing on the detectors in the experiment. That suggests that the top photon (or Alice) sees patterns and then later the bottom photon (or Bob) makes choices that affect Alice’s patterns. In both the quantum and classical experiments, only a random pattern is found on the detection screens. We have to filter those results later to see patterns. People use this imagery as shorthand for the coincidence counter and the post-experiment filtering done to the data.
- The nature of the choice is about maintaining or destroying useful information. Explanations tend to describe the “choice” very loosely like it could be a choice to go left or right, or to be dry or wet but it’s all about correlation. The photons or classical objects are correlated to begin with so the choice is about maintaining that or not. Do you want useful information or useless information? Keep the match or lose it? Do you want information you could actually use in filtering later or none so you won’t be able to do any meaningful filtering later? That’s the choice. The patterns that emerge later when you filter the full results are a reflection of what you can or can’t know about the results given your PAST choice to keep or lose the correlation. Yes the choice matters to the pattern but it’s a case of normal causality: the choice in the past influences what you will filter in the future.
I hope this was helpful. As mentioned earlier, the idea of using dice and coins came from this video on the topic and I haven’t made any discoveries or great realisations in this post. I’m just aware that I was among many who still didn’t quite get it so my aim here is to try describing it in a way that makes more sense to me. Perhaps other people might have had the same issues with the explanations as me and this may help them make more sense of it all.
I know that most reactions to this post will disagree with me and that’s ok. People are really into the retrocausality of the experiment and I was too. And of course the quantum experiment really is fascinating and raises genuinely interesting questions about the nature of quantum mechanics and how it maps onto our everyday experience. If you’re into collapsing wave functions then you’ve got lots of questions about what exactly does the collapsing and what a partial collapse means. If you’re into many worlds you’re mostly just laughing from the sidelines and wondering why everyone else is so weirded out by this experiment but it’s still interesting to ponder the differences in how decoherence occurs between single photon systems and more complex systems.
Tl;dr the delayed-choice quantum eraser experiment is a clever thought experiment turned into an impressive physical experiment. It demonstrates several interesting features of quantum mechanics and highlights the struggle to map those features onto our everyday classical experience. People think a choice later in the experiment affects events earlier in the experiment but really it’s a misunderstanding caused by several misconceptions. We are misled by people telling us it’s true; by people not being clear about when and where the patterns actually form; and us not understanding that the choice is about maintaining the ability to meaningfully filter data from the results or not being able to. We’re good at spotting patterns but bad at confusing correlation and causation. The maths and apparatus are complicated and obscure the fact that most people, myself included, simply mistake correlation for causation in the delayed-choice quantum eraser experiment.